NC General Curriculum Test Prep and Practice Questions

Resources
North Carolina General Curriculum Test Prep and Practice Questions

Welcome to our free North Carolina General Curriculum test prep and practice questions page. We’ll be introducing you to the core subtests and key concepts you need to know to pass this exam. This is one of the free resources we provide so you can see how prepared you are to take the official NC General Curriculum Test.

Quick Links to Help You Navigate This Page

NC General Curriculum Test Information
NC Multi-Subject Subtest
NC Math Subtest
NC Multi-Subject Questions and Answers
NC Math Questions and Answers

NC General Curriculum Test Information

The General Curriculum test for North Carolina assesses knowledge of each of the elementary subjects necessary for an elementary teaching license in North Carolina, including language arts, history and social science, science and technology/engineering, and mathematics.

Cost:

The Multi-Subject Subtest only is $94, the Mathematics Subtest only is $94, or if both are taken in the same appointment the test would be $139.

Scoring:

You must score at least a 227 on each subtest to pass.

Study time:

The amount of time that you will need to study depends entirely upon your existing content knowledge and your ability to retain information. We suggest using our practice test to decide which areas you need the most help on.

Once you can identify the content that you need extra help with, focus on those areas. Also, keep in mind that it’s better to spend an hour or two studying each day than to cram for the test. By using this strategy, you are more likely to retain information.

It’s a great idea to use the practice test multiple times, as well. As you review your progress, you’ll be relieved to see that your hard work is paying off!

Remember, when it comes to studying for the General Curriculum exam, there’s no such thing as knowing the content too well. Since it’s better to be safe than sorry, spend plenty of time studying. Then, you’ll be ready to test like a rockstar!

What test takers wish they would’ve known:

It is essential to arrive on time. If you are 15 minutes or more late for your exam, you will not be admitted. Plan ahead and arrive a few minutes early.
Make sure to bring your government-issued ID. If you forget to bring your ID, you will be unable to take the test.
Be sure not to bring any prohibited materials when you go to take the test. Prohibited materials include cell phones and even scratch paper. It’s not a good experience to have your score voided over an error like that!
If you have difficulty with a question, flag it and move on to one that you can answer more easily. You can return to any question at any time.
It’s better to guess than to leave a question blank! You will not be penalized for an incorrect answer, so any guess is better than no response. Return to any difficult questions that you flagged before completing the test. Eliminate any obviously incorrect choices, and guess from the rest.
It’s not wise to try to save time by skimming over the instructions. If you read carefully, you’ll avoid the risk of missing important information which could impact your score.
During written assignments, make sure that you respond to all of the assignment and read back over what you’ve written. Many test-takers catch their own mistakes that way!
Relax. It is just an exam. We’re sure that we can get you to where you need to be.

Information and screenshots obtained from: https://www.nc.nesinc.com/

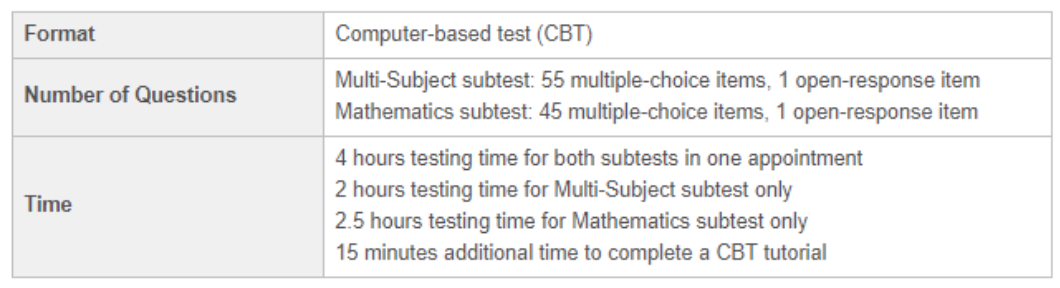
NC General Curriculum Multi-Subject Subtest

The Multi-Subject subtest has 55 multiple-choice questions that account for 90% of the exam and 1 open-response question that accounts for 10% of the exam.

This subtest can be neatly divided into 4 subareas.

Language Arts
History and Social Science
Science and Technology/Engineering
Integration of Knowledge and Understanding

So, let’s talk about them.

Language Arts

The Language Arts subarea accounts for 30% of the Multi-Subject Subtest. It includes 5 objectives. Let’s focus in on one of them.

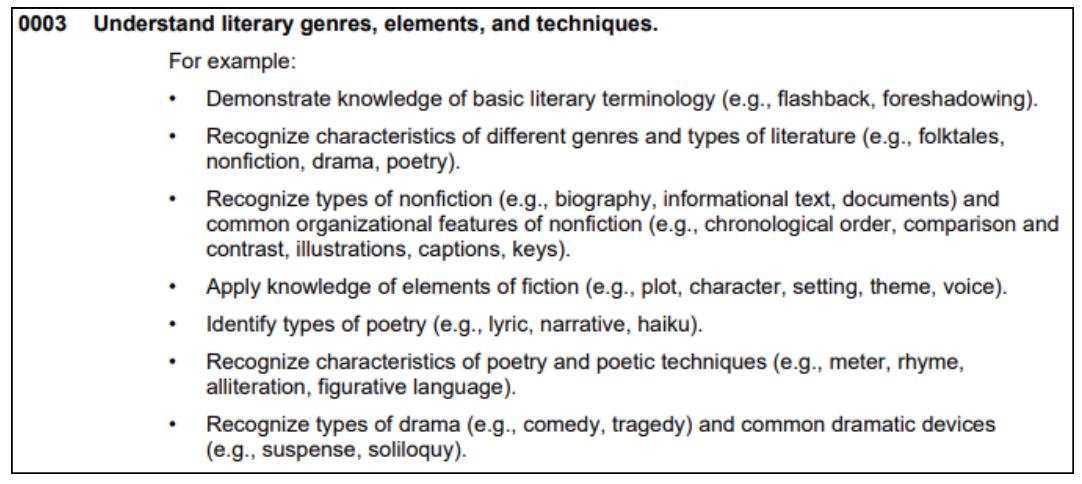
This section tests your knowledge of literary genres, elements, and techniques, including those common to fiction, nonfiction, drama, and poetry.

Let’s talk about some concepts that you will more than likely see on the test.

Genre

Literary genres are characterized by setting, plot, theme, style, tone, point of view, and characterization. Each genre is unique in one or more of those ways. Let’s focus in on specific traits about each of the following genres: folktales, drama, poetry, and nonfiction.

Folktales

Began as word of mouth stories that are passed down generation to generation.
Teach a lesson or have a moral to the story.
Creation myths are included in the folktale genre and explain how something was created or came to be.
Characters are usually people or animals.
Many contain the “rule of three”. Characters and events happen in threes.
Usually, the main character faces an impossible challenge that he or she must overcome.

Drama

Characters play a very important role in dramas.
The plot has rising and falling action, a climax, and a resolution.
There is a lot of symbolism.
There is a lot of dialogue between characters.
Usually contains a setting, theme, specific structure, and dramatic irony.

Poetry

Uses figurative language such as metaphors, similes, and allusions go beyond the literal meanings of the words and create imagery in the mind of the reader.
Three main types are lyric, narrative, and dramatic.
Poems have a particular rhyme and/or rhythm (meter).

Nonfiction

Based on real events, people, places, and events.
Does not include the writer’s imagination.
The author conveys a clear message about a specific topic.
The purpose is typically to persuade, inform, or explain.

Let’s dive in even deeper to nonfiction.

Nonfiction

Nonfiction text can include biographies, informational text, and documents.

Biographies

Biographies are non-fiction texts that detail a person’s life. A biography typically includes:

Birthdate and place of birth
Family members
Childhood and education
Interests
Interesting stories about that person’s life
Career information
Reason for fame or importance
Later life
Date and cause of death

Informational Text

Informational text primarily expresses information about science, the arts, or social studies. Informational text can be found in articles, textbooks, reference books, or digitally. The four types of informational text are:

Expository

Argument/persuasion
Procedural
Literary nonfiction

Many times informational text will have specific text features that provide the necessary information so the reader can understand the topic fully. These text features include:

Title
Table of contents
Index
Headings/subtitles
Glossary
Sidebars
Captions
Maps, charts, and graphs, keys
Photos/illustrations

Documents

A document is a written or presented thought. The word document originates from the latin word documentum, which means “teaching”. Documents have been used as “proof” throughout history. In the age we live in, we can refer to a piece of paper or a digital copy of a document that has been formatted. Documents are primarily used for:

Academics
Business
Government and law
Media

It is important to recognize the structure an author is using in a nonfiction piece because it helps the reader summarize more effectively, connect to and remember what they’ve read, and look for main ideas/important information. The five main text structures are:

Problem/solution
Cause and effect
Description/list
Sequence/chronological order
Compare/contrast

History and Social Science

The History and Social Science subarea accounts for 30% of the Multi-Subject Subtest. It includes 4 objectives. Let’s take a close look at one of them.

This section tests your knowledge of the government, founding documents, and roles of citizens in the United States. It also tests your knowledge of basic economic principles.

Here are some concepts that are likely to appear on the test:

Branches of Government

The United States government is made up of three branches. The writers of the Constitution wanted to make sure that no one person became too powerful so they created a system of checks and balances. The three branches of government are:

Executive

This branch is led by the President, but includes the Vice President, President’s cabinet, and the President’s office. The President has the power to sign a bill into law or to veto it. The laws are written by Congress, but the president has to agree to them. The executive branch also enforces laws that have been put in place by Congress. The president is the head diplomat to other countries, can pardon prisoners who have committed federal crimes, and is the head of the US Military. The President can only serve a maximum of two four-year terms.

Legislative

The legislative branch, or Congress, is made of of two parts: the Senate and the House of Representatives. This branch of government is responsible for writing and voting on laws (legislation). Congress also has the power to declare war, confirm Presidential appointments (such as Supreme Court justices), and confirm the President’s cabinet members.

The Senate has 100 members. There are two from each state.

The House of Representatives has 435 total representatives. The number from each state depends on that state’s population. The higher the population, the more representatives the state is allowed.

Judicial

The judicial branch of government is made up of judges and courts. Federal judges are appointed for life and can only be removed by death or impeachment. The reason for this is so that judges can make decisions based solely on their interpretation of the law and not have to worry about election. The judicial branch, or courts, are tasked with interpreting the laws and the Constitution.

The highest court in the United States is the Supreme Court. The Constitution does not list a specific number of justices that there should be, but there have been nine since 1869. Supreme Court justices are nominated by the President, confirmed by the Senate, and hold their offices for life.

Democratic Principles

The Declaration of Independence contained four basic principles that were reinforced and reflected in the Constitution:

All people are created equal.
All people have basic rights that cannot be taken away.
The government’s power comes from the people.
When/if the government does not protect the people, the people have the power to change or do away with the government.

Three foundations that uphold these principles are:

The Rule of Law- No person is above the law, no matter their position. This includes lawmakers themselves, law enforcement, and judges.
Due process- Due process requires that the government must respect all the legal rights that are owed to a person. Due process balances power between the laws that protect citizens and protecting an individual from the law. When due process is violated it also violates the rule of law.
Majority rule- Majority rule describes the process of voting on a decision by a 51% majority. This process is common throughout a democracy.

Science and Technology/Engineering

The Science and Technology/Engineering subarea accounts for 30% of the Multi-Subject Subtest. It includes 5 objectives. Let’s dive into one of them.

This section tests your knowledge of basic concepts and principles of the physical sciences, such as matter, energy, and simple machines.

Let’s look at some concepts that will most likely pop up on the test.

Forms of Energy

Energy is the ability to do work, can not be created or destroyed, and is constantly changing between potential and kinetic. There are five main forms of energy:

Heat (thermal) energy results from atoms or molecules moving in solids, liquids, and gases and can be transferred between objects.
Electrical energy results from the flow of an electric charge.
Magnetic energy is the potential energy stored in a magnetic field.
Sound energy is a type of mechanical energy. It is produced when sound vibrations cause waves that travel through solids, liquids, or gases.
Light energy is the only form of energy that can be seen directly. We are able to see different colors of light because each color has a different wavelength. Every color we see is either on the color spectrum or is a combination of colors on the spectrum (red, orange, yellow, green, blue, indigo, violet).

Motion

Motion is a change in an object’s position over time. Two important terms related to motion are inertia

and momentum.

Inertia is best described by Newton’s First Law of Motion, which states: an object in motion or rest will stay in that state unless acted upon by an outside force. For example, it is so important to wear a seat belt in a car because when your car is going 60 miles per hour, so are you. If your car stops immediately, a force acts on the car. Without a seat belt, you would continue to move 60 miles per hour. Your seatbelt acts as the force to change your motion.

Momentum is basically an object’s mass in motion. All objects have mass, so an object that is moving has momentum. Momentum can be calculated by multiplying an object’s mass (how much of the object there is) by its velocity (how fast the object is moving).

Momentum= Mass x Velocity

Integration of Knowledge and Understanding

The Integration of Knowledge and Understanding subarea accounts for 10% of the Multi-Subject Subtest. It is a written response to a question that covers content in the following objective.

This section tests your ability to prepare an organized and developed analysis on a topic.

The open-ended response section of the test is scored on four areas. Scores look for how clearly and effectively you communicate:

Purpose
Subject matter knowledge
Supporting evidence
Rationale

To receive full credit for your response, be sure to:

Fully answer the question given.
Apply subject matter knowledge substantially, accurately, and appropriately.
Use sound and high-quality supporting evidence in your writing that includes relevant examples.
Respond in a way that demonstrates a comprehensive and well reasoned understanding of the topic.

This section of the test is meant to assess your subject area knowledge, and reference materials cannot be used. Your response is expected to be between 150-300 words, or 1-2 pages. It should demonstrate the depth of your understanding of the subject, rather than just listing facts.

Take a look at the following link to see a strong example of this type of written response:

https://www.nc.nesinc.com/Content/STUDYGUIDE/SA_SG_CRI_GC.htm#responses

And that’s some basic info about the NC General Curriculum Multi-Subject subtest.

NC General Curriculum Math Subtest

The Mathematics subtest test has 45 multiple-choice questions that account for 90% of the exam and 1 open-response question that accounts for 10% of the exam.

This subtest can be neatly divided into 5 subareas.

Numbers and Operations
Functions and Algebra
Geometry and Measurement
Statistics and Probability
Integration of Knowledge and Understanding

So, let’s discuss them.

Numbers and Operations

The Numbers and Operations subarea accounts for 41% of the Mathematics Subtest. It includes 4 objectives. Let’s look at one closely.

This section tests your knowledge of mathematical operations, including fluently understanding and applying rules of operations, exponents, and fractions.

Let’s talk about some concepts that you will more than likely see on the test.

Arithmetic Properties

Arithmetic properties are rules or ways of thinking that can be used when performing basic mathematical operations, such as addition, subtraction, multiplication, and division.

Commutative Property

“Commutative” comes from the word “commute”, or move around. This property refers to changing the order of numbers when multiplying or adding them, but finding the same answer. For example:

Addition: b + c = c + b or 8 + 7 = 7 + 8

Multiplication: cb = bc or 7 x 8 = 8 x 7

Associative Property

“Associative” comes from the word “group.” This property refers to how numbers are grouped. If the grouping of numbers has changed, the associative property has been used. For example:

Addition: a + (b + c) = (a + b) + c or 3 + (2 + 1) = (3 + 2) + 1

Multiplication: (ab)c = a(bc) or (3 x 2)1 = 3(2 x 1)

Distributive Property

The distributive property is used anytime a number is “multiplied” or factored out through parentheses. For example:

a (b + c) = ab + ac or 3(2 + 4) = (3 x 2) + (3 x 4)

Each of these properties is related to the computational algorithm. The algorithm is a sequence of steps that solve a problem, and these properties support the algorithm.

The properties are also important when performing mental math calculations because they allow for some liberties in combining numbers to make groups of 10 or common multiples. This aids in mental math.

Order of Operations

The order of operations refers to the steps necessary to solve multi-operation numerical expressions. The order of operations is PEMDAS:

P- Solve any operations within parentheses while still using the order of operations for multiple operations.
E- Solve for exponents.
M/D- Solve all multiplication OR division in order left to right.
A/S- Solve all addition OR subtraction in order left to right.

For example, to evaluate

8 + (2 x 5) x 3³ ÷9:

8 + (2 x 5) x 3³ ÷9

Perform operations in parentheses: 2 x 5 = 10

8 + 10 x 3³ ÷9

Solve for exponents: 3³ = 27

8 + 10 x 27 ÷9

Solve for multiplication OR division left to right:10 x 27 = 270, then 270÷ 9 = 30

8 + 270 ÷9

8 + 30

Solve for addition OR subtraction left to right: 8 + 30 = 38

The answer is 38 since there are no more operations to complete.

Functions and Algebra

The Functions and Algebra subarea accounts for 22% of the Mathematics Subtest. It includes 3 objectives. Let’s talk one through.

This section tests your ability to understand and solve algebraic expressions.

Let’s look at some concepts that will most likely pop up on the test.

Algebraic Expressions

Algebraic expressions are similar to numerical expressions, except they include at least one variable and at least one operation, but do not include an equal sign. That would be an algebraic equation. For example, a very simple algebraic expression would be:

2(x + 4) + 3(y – 5) – 2y

Use the distributive property to eliminate the parentheses

2x + 8 + 3y – 15 – 2y

Rewrite like terms together (3y and -2y as well as 8 and -15)

3y – 2y + 8 – 15 + 2x

Combine like terms (3y-2y=y and 8-15= -7)

y + 2x – 7

Ratios

A ratio is a relationship between numbers that indicates how many times the first number contains the second number. For example, if a bowl of trail mix contains 1 cup of chocolate candies and 3 cups of peanuts, the ratio of chocolate candies to peanuts is 1 to 3 or 1:3.

A ratio problem might look like this:

A jar contains 72 marbles. 15 are blue, 42 are red, and the rest are green. What is the ratio of blue marbles to green marbles?

To solve:

Figure out how many green marbles there are in the jar by adding 15(blue)+42 (red) then subtract that sum (57) from 72 (the total number of marbles) because we know that the rest of the marbles must be green. 72-57=15, so we know there are 15 green marbles.
Now compare the number of blue marbles to green marbles. There are 15 blue and 15 green marbles, so the relationship is 15 to 15 or 15:15. Just like a fraction, 15 can be simplified.
Simplify 15:15 by dividing both by their greatest common factor (15)
The answer is 1 to 1 or 1:1

Geometry and Measurement

The Geometry and Measurement subarea accounts for 18% of the Mathematics Subtest. It includes 2 objectives. Let’s take a close look at one.

This section tests your ability to understand and apply concepts of geometry, including analysis of 2 and 3-dimensional figures, transformations, and concepts related to algebra.

Here are some topics that are likely to appear on the test:

Attributes

Polygons

A polygon is a two-dimensional, closed figure comprised of line segments and vertices. Polygons can be classified by attributes, including the number of sides and measures of internal angles. Types of polygons include:

3 sided polygon: triangle
4 sided polygon: quadrilateral
5 sided polygon: pentagon
6 sided polygon: hexagon
7 sided polygon: heptagon
8 sided polygon: octagon
9 sided polygon: nonagon
10 sided polygon: decagon

A polygon that is made up of congruent sides and angles is called a regular polygon. For example, a five sided figure that is made up of five congruent sides and angles would be classified as a regular pentagon.

Triangles and quadrilaterals have unique classifications based on the measurements of their sides and angles.

Triangles can be classified by sides:

Equilateral- 3 congruent sides
Isosceles- 2 congruent sides
Scalene- no congruent sides

Or by angles:

Acute- all angles measure less than 90 degrees
Obtuse- one angle measures greater than 90 degrees
Right- one angle measures 90 degrees

A quadrilateral can be a:

Parallelogram- two pairs of parallel sides
Trapezoid- one pair of parallel sides
Square- have all congruent sides and four right angles
Rectangle- have two sets of congruent sides and four right angles

Three Dimensional Figures

Three dimensional figures have a third dimension (depth). Three dimensional figures include:

Prisms
Cubes
Spheres
Cones
Cylinders

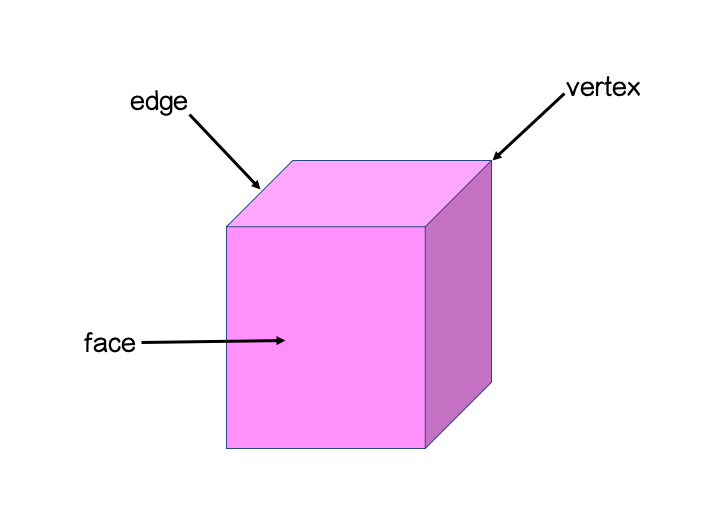
Similar to 2D polygons, 3D figures can be classified by their attributes. These attributes include faces, vertices, and edges.

Face- a two dimensional shape that makes up one flat surface of a 3D shape
Edge- where two faces meet
Vertex (vertices)- the point or corner of the shape

3d shape

Geometric Transformations

An image can be changed using different operations or transformations. The most common transformations are:

Translation- The figure’s position changes and appears to have slid in any direction.
Rotation- The figure rotates around a fixed point.
Reflection- The figure is flipped over a line.
Dilation- The figure’s size is enlarged or reduced.

For more information on geometric transformations, check out this resource: https://www.mathsisfun.com/geometry/transformations.html

Statistics and Probability

The Statistics and Probability subarea accounts for 9% of the Mathematics Subtest. It includes 2 objectives. Let’s discuss one.

This section tests your ability to interpret and describe data and statistical information.

Here is a concept that is likely to appear on the test:

Measures of Central Tendency

The measure of central tendency is the typical value for a probability distribution. Measures of central tendency include:

Mean

This is the most popular measure. It is the average or norm or a data set. The mean is found by finding the sum of all numbers in a set then dividing the sum by how many numbers there are in the set.

Example: In the set 2, 2, 3, 5, 5, 5, 7, 8, then mean is 5.29. 2+2+3+5+5+5+7+8=37. 37 divided by 7 (the total number of values in the set) equals 5.29.

Median

This is the middle value of a data set when the numbers are ordered from least to greatest.

Example: In the set 2, 2, 3, 5, 5, 7, 8, the median is five. If there is an even number of values in the data set, you average the middle two to find the median by adding them together and dividing by two.

Mode

This is the most frequent value in a data set. To find the mode, list all numbers in the set from least to greatest, then find the number that appears most frequently.

Example: In the set 2, 2, 3, 5, 5, 7, 8, the number 5 appears most frequently, so the mode is 5. If more than one number appears the most frequently, then each of those numbers would be included in the mode. If no numbers in the set appear more than once, then there is no mode.

Spread

The simplest way to find the spread in a data set is to find the range which is the difference between the least and greatest number in the set.

Example: In the set 2, 2, 3, 5, 5, 7, 8, the spread is six. The greatest number in the set is 8, and the number of least value in the set is 2. The difference between 8 and 2 is 6.

Integration of Knowledge and Understanding

The Integration of Knowledge and Understanding subarea accounts for 10% of the Mathematics Subtest. It is a written response to a question that covers content in the following objective.

This section tests your ability to integrate your knowledge and understanding of mathematics and communicate multiple solutions to a problem in detail.

The open-ended response section of the test is scored on four areas. Scores look for how clearly and effectively you communicate:

Purpose
Subject matter knowledge
Supporting evidence
Rationale

To receive full credit for your response, be sure to:

Fully answer the question given.
Apply subject matter knowledge substantially, accurately, and appropriately.
Use sound and high-quality supporting evidence in your writing that includes relevant examples.
Respond in a way that demonstrates a comprehensive and well reasoned understanding of the topic.

In this section, you will have to use your specific knowledge of math to create a response in which you analyze the student’s work and provide an alternative solution to the problem. Your response should:

Correct any errors or misconceptions shown in the student’s work AND explain why the response is not correct. Be sure to:

Include the correct solution
Show your work
Explain your reasoning

Also, it is important to solve the problem using an alternative method that could help the students conceptual understanding of the topic in the next problem.

The following provides an example of a question and strong response to this type of prompt: https://www.nc.nesinc.com/Content/STUDYGUIDE/SA_SG_CRI_GC.htm#responses

And that’s some basic info about the Mathematics subtest of the NC General Curriculum Exam.

NC General Curriculum Multi-Subject Practice Questions

Question 1

Mrs. Landingham has noticed that her students are great at identifying similes in their reading, but she wants to know if the students understand what they mean. What would be the best way for her to evaluate the students’ understanding of similes they identified?

Ask them to explain what two things are being compared and what they have in common.
Ask them to write their own similes.
Ask them to explain how it is different than a metaphor.
Ask them to rewrite the sentence that contains a simile without figurative language.

Correct Answer: 1.

Explanations:

This will help students explain why an author used a simile in their writing and show whether the student understands the meaning of the figurative language.
Writing your own similes is a great creative writing activity, but does not demonstrate understanding about a particular similes’ purpose in a text.
Comparing similes and metaphors will help students better understand their differences, but does not demonstrate understanding about a particular simile’s purpose in a text.
Removing the figurative language would not demonstrate the understanding of the similes’ meaning.

Question 2

Read the introductory paragraph from a student’s compare/contrast essay below and answer the question that follows.

(1) Apples and oranges are two delicious foods. (2) Apples and oranges grow on trees. (3) Apples and oranges are considered fruits, not vegetables. (4) I like apples more than oranges. (5) Even though apples and oranges look different on the outside, they have a lot of traits in common.

Which sentence should be removed from the paragraph to maintain clarity and purpose?

Sentence 1
Sentence 2
Sentence 4
Sentence 5

Correct Answer: 3

Explanations:

Sentence 1 is a topic sentence and should be included.
Sentence 2 previews the main point of comparison between the fruits.
Given that the prompt indicates this is a compare/contrast essay sample, the best sentence to remove would be Sentence 4. The student’s opinion on which fruit is best is unnecessary information for this type of essay. An introductory paragraph should focus on previewing what facts and details will be discussed later in the essay.
Sentence 5 is the thesis statement and should not be removed.

Question 3

When students are given two texts on the same subject, one persuasive and one informative, what approach would be most helpful to determining purpose?

Determine whether each topic sentence is a claim or factual statement.
Summarize each paragraph in the margin and identify each paragraph’s main idea.
Evaluate the author’s use of citations and verify that the sources are reliable.
Evaluate the author’s use of figurative language.

Correct Answer: 1.

The topic sentences indicate the goal of the paragraph. If it is making an argumentative claim, the reader will know the author intends to persuade. If it is a factual statement, the reader will know the author’s intention is to inform.

Question 4

Thomas Jefferson purchased the Louisiana Territory from:

Britain
Russia
Spain.
France.

Correct Answer: 4.

Thomas Jefferson purchased the Louisiana Territory from France in 1803 for the price of $15 million or roughly three cents per acre. The purchase doubled the size of the United States overnight. Napoleon sold the land to finance his European warfront. The purchase included almost all the land between the Mississippi River and the Rocky Mountains, with the exception of modern-day Texas.

Question 5

According to the constitution, which of the following does NOT describe a purpose of the United States government?

establishing democratic governments abroad
regulating voting and elections in the United States
providing public services to the people of the United States
maintaining defense for the United States and its citizens

Correct Answer: 1.

The U.S. government may act as an advocate for democratic principles around the world, but it is not tasked with establishing new democratic governments.

Question 6

Which of the following describes some of the largest Roman contributions to Western society?

advancements in geometry
the organization of the central government and written laws
the first known novels, Homer’s the Iliad and the Odyssey
the Greek language

Correct Answer: 2.

The Roman advancements in political science, from the structure to the administration of government, provided a framework for the Founding Fathers of the United States

Question 7

Which of the following is the best example of a country’s location affecting its natural resources?

Great Britain’s ten major ports helped the country become a center for international banking.
The small size of Singapore has led to one of the highest population densities in the world.
The many climate zones of the United States allow for wide agricultural diversity and high yields of crops.
Australia’s proximity to China has been beneficial for its export markets to that country.

Correct Answer: 3.

Explanations:

Though the location of so many large ports in Great Britain has affected how its people live, this is not an example of natural resources.
While the location of Singapore on a small island in Malaysia has affected how people live, encouraging very dense development on the limited land, this is not an example of natural resources.
The location of the United States allows for many climate zones, which affect its natural resources.
While Australia’s location near China has affected trade between the two countries, this is not an example of natural resources.

Question 8

Which of the following gives the correct equation for photosynthesis?

oxygen + water + light energy -> carbon dioxide + glucose
carbon dioxide + water + light energy -> oxygen + glucose
oxygen + glucose -> carbon dioxide + water + energy
carbon dioxide + glucose -> oxygen + water + energy

Correct Answer: 2.

Photosynthesis uses carbon dioxide, water, and light energy to produce oxygen and glucose.

Question 9

A teacher is teaching a unit on weather to the class. As a demonstration, the teacher heats a beaker on a hot plate and adds blue food coloring to the water. The students observe the movement of coloring in the water. Which of the following processes is best demonstrated by the movement of the food coloring?

Convection
Conduction
Evaporation
Radiation

Correct Answer: 1.

Explanations:

Convection is the process of heat transfer by currents in fluids, which is observed in the teacher’s experiment.
Conduction involves the transfer of heat by the exchange of energy between colliding particles, which is not observed in the teacher’s demonstration.
Evaporation is the process of liquid on the surface of an object evaporating into a gas. This is not observed in the teacher’s demonstration.
Radiation is the process of energy transfer by electromagnetic waves. This is not observed in the teacher’s demonstration.

Question 10

A local farmer cuts down a population of trees next to a river so that he can plant a field of crops. Which of the following will be the most likely result because of the loss of trees next to the river?

an increase of natural waste into the river
an increase in wildlife population along the river
a decrease in the amount of water in the river
an increase of soil erosion into the river

Correct Answer: 4.

Explanations:

Trees, and the wildlife that lives in forest, would contribute a large amount of natural waste into the river through the form of excrement and old vegetation. Without the trees and wildlife, the amount of natural waste in the river would most likely decrease.
A decrease in trees would reduce the amount of food and shelter in the area. This would most likely reduce wildlife population along the river because much of the food and shelter for the wildlife is removed.
There is no reason cutting down trees would decrease the amount of water in the river.
Without the trees and other vegetation near the river, the erosion of the soil would most likely increase. Vegetation, such as trees and shrubs, helps reduce soil erosion because of the root systems and natural barriers that prevent erosion of the soil. With the trees removed, there are less barriers for the soil to erode into the river.

NC General Curriculum Math Practice Questions

Question 1

Which of the following is not a rational number?

-9.86398
Π
0
843

Correct Answer: 2.

Explanations:

Rational numbers can be written as an exact fraction and decimal, so this number is rational.
The value of pi (π) never ends and does not repeat, making it irrational.
Rational numbers can be written as an exact fraction and decimal, so this number is rational.
Rational numbers can be written as an exact fraction and decimal, so this number is rational.

Question 2

Team 1 scored 31.25% of the points. 0.375 of the points were scored by Team 2. Team 3 scored 3/16 of the points and 1/8 of the points scored were by Team 4.

What percentage of the points were scored by even numbered teams?

50
0.5
68.8
31.25

Correct Answer: 1.

Explanations:

The even numbered teams are 2 and 4. Team 2 scored 0.375 of the total points which converts to 37.5%. Team 4 scored 1/8 of the points which is 0.125 as a decimal and 12.5% as a fraction. 37.5 + 12.5 = 50
0.5 is correct, but the question asked for the answer to be a percentage. When converted, 0.5 = 50%.
This is the total percentage of Teams 1 and 2.
This is the percentage for Team 1.

Question 3

Mr. Harris assigns his students to create a factor tree of the products of a number. One student turns in the product factor tree of 32 shown here. Which of the following best describes the error in this factor tree?

The factor tree does not identify the factors of 2.
The factor tree does not correctly identify the factors of 32.
The factor tree identifies the sums and not the product factors of many of numbers.
There is no error in the tree.

Correct Answer: 3.

Explanations:

2 is prime and does not need to be factored.
8 and 4 are factors of 32.
The third level of factors is the sum of the second level numbers (4 + 4 = 8). The correct factors of eight would be either (4 and 2) or (1 and 8).
The errors are at the third level of the tree

Question 4

Which of the following requires the most advanced understanding of relationships between arithmetic operations?

47 + 53 = 50 + 50 = 100
53 – 47 + 4 = 57 – 47 = 10
(4 × 3) + (4 × 3 – 2) = (12) + (12 – 2) = 22
100 – 36 = 64

Correct Answer: 3.

Explanations:

This is rounding and basic addition. It does not require advanced thinking.
This uses the commutative property of addition, but this could be solved without knowledge of properties.
This requires the knowledge of the Order of Operations and multiplication rules.
This is basic subtraction which does not require high-level thinking or understanding.

Question 5

Which of the following comparisons between equations and inequalities is NOT true?

Equations typically have only one solution that makes the equation true, while inequalities typically have a solution set, or range of values, that make the inequality true.
An equation is a statement that two expressions are equal, while an inequality is a statement that two expressions are not equal.
Equations contain only one possible symbol between each expression (=), while inequalities can contain four possible symbols between each expression (<, >, ≤, ≥).
Equations are solved using inverse operations while inequalities cannot be solved with inverse operations.

Correct Answer: 4.

Explanations:

This is true; equations typically have only 1 value that makes the equation true (x+3=4; x=1 is the only value for x the makes the equation true), while inequalities typically have a range of values that make the inequality true (x+3>4; x>1 meaning 2, 3, 4, 5, 6, etc. all make the inequality true).
This is true; equations are 2 expressions that are equal, and inequalities are 2 expressions that are not equal.
This is true; equations only contain = between the 2 expressions, while inequalities can have <, >, ≤, or ≥ between the expressions.
This is false; both equations and inequalities can be solved using inverse operations to isolate the variable.

Question 6

Isabella is creating a function in the form of a table of values. So far, her table looks as follows:

Isabella is creating a function in the form of a table of values. So far, her table looks as follows:

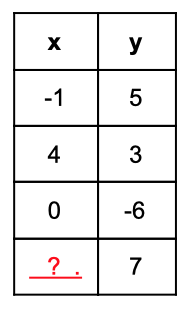
Which of the following numbers could

not

be substituted into the blank in the table and still have the relation represent a function?

7
3
-1
1

Correct Answer: 3.

Explanations:

Adding 7 as an input would still follow the rule of each input only corresponding with one output, so the relationship still represents a function. Even though the input and output are the same, it is still a function.
Adding a 3 as an input still follows the rule of each input only having one output, so the relation is still a function.
There is already an input of -1 in the table, which corresponds to the output of 5. Inserting another input of -1 that corresponds to a different output of 7 makes the relation no longer a function, because each input does not have only 1 corresponding output. The input of -1 now has 2 outputs, 5 and 7.
Inserting 1 as an input still follows the rule of each input only having one output, and so the relation would still be a function. Although there is already an input of negative 1, positive 1 is a different value and so is a different input.

Correct Answer: 3.

Explanations:

Adding 7 as an input would still follow the rule of each input only corresponding with one output, so the relationship still represents a function. Even though the input and output are the same, it is still a function.
Adding a 3 as an input still follows the rule of each input only having one output, so the relation is still a function.
There is already an input of -1 in the table, which corresponds to the output of 5. Inserting another input of -1 that corresponds to a different output of 7 makes the relation no longer a function, because each input does not have only 1 corresponding output. The input of -1 now has 2 outputs, 5 and 7.
Inserting 1 as an input still follows the rule of each input only having one output, and so the relation would still be a function. Although there is already an input of negative 1, positive 1 is a different value and so is a different input.

Question 7

What is the area, in square units, of the right trapezoid above?

168
120
216
180

Correct Answer: 1.

The area of a trapezoid can be found using the formula A = 1/2(b1 + b2)h, where A is the area, b1 and b2 are the lengths of the bases of the trapezoid (the pair of parallel sides), and h is the height of the trapezoid. In this case, the bases have lengths of 18 and 10. Because the given information in the question states that the figure is a right trapezoid, the height of the trapezoid is known to be 12. Therefore, the area of this right trapezoid is A = 1/2(18 + 10)12 = 1/2(28)(12) = 14 × 12 = 168 square units.

Another way to address the question is to notice that the right trapezoid in the diagram is composed of a 12 × 10 unit rectangle connected to a right triangle with a length of 12 units and a height of 18 – 10 = 8 units. (It may help to sketch in a line segment at the top of the side that measures 10 units and parallel to the side that measures 12 units until the segment meets the line that measures 18 units. That line segment will measure 12 units, and will split the 18 unit long side into a 10 unit portion and an 8 unit portion, dividing the trapezoid into two more common shapes, the rectangle and right triangle.) The area of the rectangle and the triangle can be calculated and then added together to get the total area of the trapezoid.

The area of a rectangle is found with the formula A = bh or A = lw, where A is the area, b is the base, h is the height, l is the length, and w is the width. In this case, the dimensions of the rectangle are 12 × 10. Therefore, the area of the rectangle is 12 × 10 = 120 square units.

The area of a triangle is found with the formula A = 1/2bh, where A is the area, b is the base, and h is the height of the triangle. In this case, the dimensions of the triangle are 12 × 8. Therefore, the area of the triangle is 1/2(12)(8) = 6 × 8 = 48 square units.

The total area of the trapezoid is 120 + 48 = 168 square units.

Question 8

If ∆DEF, shown in the xy-plane above, were to be shifted 5 units to the left and 6 units up, which of the following would be the coordinates of point E after the translation?

(-2, 11)
(8, 11)
(9, 0)
(-3, 10)

Correct Answer: 1. A translation of a geometric figure moves the entire figure to a new location in the same coordinate plane. If the movement is a shift to the left, the x-coordinate (first coordinate) should be decreased. In this case, a shift to the left of 5 units results in subtracting 3 – 5 and yields a new x-coordinate of -2. If the movement includes a shift up, the y-coordinate (second coordinate) should be increased. In this case, a shift up of 6 units results in adding 5 + 6 and yields a new y-coordinate of 11. In other words, if the point (3, 5) is shifted 5 units to the left and 6 units up, the new point will be (3 – 5, 5 + 6) = (-2, 11).

Question 9

Edward wants to make a plot that depicts his classmate’s favorite types of juice. Which of the following plots would be best suited for that?

Histogram
Pie Chart
Scatterplot
Stem-and-Leaf plot

Correct Answer: 2.

Explanations:

Histograms are best used for plotting large amounts of numerical data. Favorite type of juice is categorical, not quantitative.
Pie charts are useful for plotting categorical data.
Scatterplots are useful for plotting two sets of numerical data against each other. Favorite type of juice is categorical, not quantitative.
Stem-and-leaf plots are useful for quantitative not categorical data.

Question 10

In the pair of dice that Tim rolled 25 times, he recorded a sum of 4 on three of those rolls. What is the difference between the theoretical probability and the experimental probability of rolling a pair of dice and getting a sum of 4 based on Tim’s experiment?

The experimental probability is about 4% greater than the theoretical probability
The theoretical probability is about 8% less than the experimental probability
The experimental probability and the theoretical probability are the same
The experimental probability is 3/25, but we don’t know the theoretical probability for Tim’s simulation

Correct Answer: 1. Theoretical probability is determined by analyzing the situation, in this case the rolling of a pair of dice, and determining the number of successful outcomes divided by the number of possible outcomes. The experimental probability is the probability that occurred in this specific experiment. The theoretical probability of rolling a sum of 4 is PT(4) = 3/36 = 1/12, about 8.3%. When Tim rolled his dice 25 times, he got a 4 on three of those rolls. While the theoretical probability of rolling a pair of dice and getting a sum of 4 is always about 8.3%, the probability that occurred in Tim’s experiment, the experimental probability, is PE(4) = 3/25 = 0.12 = 12%. This means his experimental probability was about 4% greater than the theoretical probability (12% – 8.3% = 3.7%, about 4%).